报 告 题 目：Liouville-type theorems and existence of solutions for quasilinear elliptic equations with nonlinear gradient terms

报 告 人：张正策 教授

报 告 时 间：2024年11月14日上午10:00

地点：太原理工大学明向校区数学学院602

内容简介：

In this talk, we consider two properties of positive weak solutions of quasilinear elliptic equation 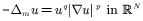, with nonlinear gradient terms. First, we show a Liouville-type theorem for positive weak solutions of the equation involving the m -Laplacian operator. The technique of Bernstein gradient estimates is ultilized to study the case p<m. Moreover, a Liouville-type theorem for supersolutions under subcritical range of exponents  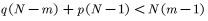is also established. Then, we use a degree argument to obtain the existence of positive weak solutions for a nonlinear Dirichlet problem of the type 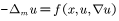, with f satisfying certain structure conditions. Our proof is based on a priori estimates, which will be accomplished by using a blow-up argument together with the Liouville-type theorem in the half-space. As another application, some new Harnack inequalities are proved. This is a joint work with Caihong Chang and Bei Hu.

报告人简介：

张正策，2003年博士毕业于西安交通大学理学院，现任西安交通大学数学与统计学院教授，博士生导师，从事非线性偏微分方程理论及其应用研究。近年来, 主要对非线性抛物方程的梯度爆破和自由边界问题开展定性研究，主持国家自然科学基金和省部级基金多项，在国际学术刊物CVPDE, JDE, DCDS, Siam J Numer Anal, NA等发表论文80余篇。多次应邀参加CMSIC, AIMS和AMS Spring Section等国际学术会议并作报告，担任美国数学会评论员。